Python值pandas包调用scatter_matrix出错
本文共 116 字,大约阅读时间需要 1 分钟。

import pandas as pdprint(pd.__version__)
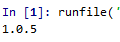
pd.plotting.scatter_matrix
转载地址:http://sgqx.baihongyu.com/
你可能感兴趣的文章
MySQL灵魂拷问:36题带你面试通关
查看>>
mysql状态分析之show global status
查看>>
mysql状态查看 QPS/TPS/缓存命中率查看
查看>>
mysql生成树形数据_mysql 实现树形的遍历
查看>>
mysql用于检索的关键字_Mysql全文搜索match...against的用法
查看>>
MySQL用得好好的,为什么要转ES?
查看>>
MySql用户以及权限的管理。
查看>>
MySQL用户权限配置:精细控制和远程访问的艺术!------文章最后有惊喜哦。
查看>>
mysql用户管理、常用语句、数据分备份恢复
查看>>
MySQL留疑问:left join时选on还是where?
查看>>
mysql登陆慢问题解决
查看>>
Mysql百万级数据查询优化
查看>>
MySQL的 DDL和DML和DQL的基本语法
查看>>
mysql的 if else , case when then, IFNULL
查看>>
MySQL的10种常用数据类型
查看>>
MySQL的btree索引和hash索引的区别
查看>>
mysql的cast函数
查看>>
MySql的CRUD(增、删、改、查)操作
查看>>
MySQL的DATE_FORMAT()函数将Date转为字符串
查看>>
mysql的decimal与Java的BigDecimal用法
查看>>